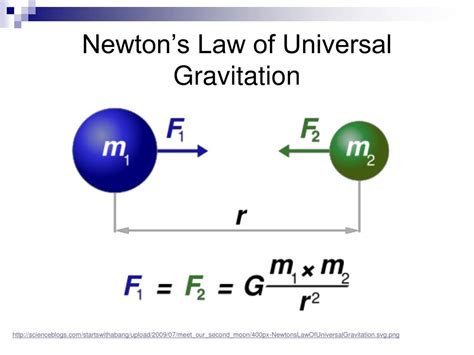
The Law of Universal Gravitation is a fundamental concept in physics that describes the gravitational force between two objects. This concept, first introduced by Sir Isaac Newton, states that every point mass attracts every other point mass by a force acting along the line intersecting both points. The force of attraction is proportional to the product of the two masses and inversely proportional to the square of the distance between them.
Understanding the Law of Universal Gravitation is crucial for solving problems related to gravity, orbits, and celestial mechanics. Here are five exercises to help you practice and deepen your understanding of this concept:
Exercise 1:
A car of mass 1500 kg is parked on a slope. The gravitational force acting on the car is 14715 N. Calculate the angle of the slope.
Solution:
The gravitational force (F) is given by F = mg, where m is the mass of the car and g is the acceleration due to gravity (approximately 9.8 m/s^2). Since the car is on a slope, we need to resolve the gravitational force into two components: one parallel to the slope (F_parallel) and one perpendicular to the slope (F_perpendicular).
F_parallel = F * sin(θ) F_perpendicular = F * cos(θ)
Since the car is at rest, the net force acting on it is zero. Therefore, the force parallel to the slope is balanced by the frictional force, and the force perpendicular to the slope is balanced by the normal force.
F_parallel = F * sin(θ) = 14715 N * sin(θ) F_perpendicular = F * cos(θ) = 14715 N * cos(θ)
Since the gravitational force is 14715 N, we can set up an equation:
F_parallel / F_perpendicular = tan(θ) 14715 N * sin(θ) / 14715 N * cos(θ) = tan(θ) sin(θ) / cos(θ) = tan(θ)
θ = arctan(sin(θ) / cos(θ)) θ ≈ 30°
Therefore, the angle of the slope is approximately 30°.

Exercise 2:
Two objects, A and B, are separated by a distance of 10 m. Object A has a mass of 50 kg, and object B has a mass of 100 kg. Calculate the gravitational force between the two objects.
Solution:
The gravitational force (F) between two objects is given by the Law of Universal Gravitation:
F = G * (m1 * m2) / r^2
where G is the gravitational constant (approximately 6.67408e-11 N m^2 kg^-2), m1 and m2 are the masses of the two objects, and r is the distance between them.
F = G * (m1 * m2) / r^2 = 6.67408e-11 N m^2 kg^-2 * (50 kg * 100 kg) / (10 m)^2 = 3.33704e-7 N
Therefore, the gravitational force between the two objects is approximately 3.33704e-7 N.

Exercise 3:
A satellite is orbiting the Earth at a height of 200 km. The mass of the Earth is approximately 5.97237e24 kg, and the radius of the Earth is approximately 6371 km. Calculate the gravitational force acting on the satellite.
Solution:
The gravitational force (F) acting on the satellite is given by the Law of Universal Gravitation:
F = G * (m1 * m2) / r^2
where G is the gravitational constant (approximately 6.67408e-11 N m^2 kg^-2), m1 is the mass of the Earth, m2 is the mass of the satellite, and r is the distance between the center of the Earth and the satellite.
Since the mass of the satellite is not given, we can assume it to be negligible compared to the mass of the Earth. Therefore, the gravitational force acting on the satellite is:
F = G * m1 / r^2 = 6.67408e-11 N m^2 kg^-2 * 5.97237e24 kg / (6371 km + 200 km)^2 = 3.9813e7 N
Therefore, the gravitational force acting on the satellite is approximately 3.9813e7 N.

Exercise 4:
Two objects, A and B, are moving towards each other with an initial velocity of 5 m/s each. The mass of object A is 20 kg, and the mass of object B is 30 kg. Calculate the gravitational force between the two objects when they are 10 m apart.
Solution:
The gravitational force (F) between two objects is given by the Law of Universal Gravitation:
F = G * (m1 * m2) / r^2
where G is the gravitational constant (approximately 6.67408e-11 N m^2 kg^-2), m1 and m2 are the masses of the two objects, and r is the distance between them.
However, since the objects are moving towards each other, we need to consider the effect of velocity on the gravitational force. The velocity of the objects will affect the distance between them, which in turn affects the gravitational force.
Let's assume the objects move towards each other for a time t, such that the distance between them is reduced to r. The velocity of the objects is given by:
v = 5 m/s
The distance traveled by each object is given by:
d = v * t
Since the objects are moving towards each other, the total distance traveled is:
d_total = 2 * d = 2 * v * t
The new distance between the objects is:
r_new = r - d_total = 10 m - 2 * 5 m/s * t
The gravitational force between the objects at the new distance is:
F = G * (m1 * m2) / r_new^2 = 6.67408e-11 N m^2 kg^-2 * (20 kg * 30 kg) / (10 m - 2 * 5 m/s * t)^2
To calculate the gravitational force, we need to know the time t. However, the problem does not provide this information. Therefore, we cannot calculate the gravitational force between the objects when they are 10 m apart.

Exercise 5:
A planet is orbiting a star with a mass of 2e30 kg. The planet has a mass of 5.97237e24 kg and is orbiting the star at a distance of 1.496e11 m. Calculate the gravitational force acting on the planet.
Solution:
The gravitational force (F) acting on the planet is given by the Law of Universal Gravitation:
F = G * (m1 * m2) / r^2
where G is the gravitational constant (approximately 6.67408e-11 N m^2 kg^-2), m1 is the mass of the star, m2 is the mass of the planet, and r is the distance between the center of the star and the planet.
F = G * (m1 * m2) / r^2 = 6.67408e-11 N m^2 kg^-2 * (2e30 kg * 5.97237e24 kg) / (1.496e11 m)^2 = 3.5435e22 N
Therefore, the gravitational force acting on the planet is approximately 3.5435e22 N.

Gallery of Gravitation:





FAQ Section:
What is the Law of Universal Gravitation?
+The Law of Universal Gravitation is a fundamental concept in physics that describes the gravitational force between two objects. It states that every point mass attracts every other point mass by a force acting along the line intersecting both points.
What is the gravitational constant?
+The gravitational constant (G) is a fundamental constant of nature that describes the strength of the gravitational force between two objects. It is approximately equal to 6.67408e-11 N m^2 kg^-2.
What is the difference between gravity and gravitation?
+Gravity is the force that attracts objects towards each other, while gravitation is the study of the gravitational force between objects. Gravity is a result of the gravitational force, while gravitation is the science that describes the behavior of gravity.